Solvation energy difference in two-immiscible electrolyte
Figure 1 explains the working principle of how the cation solvation switching in two-immiscible electrolyte induces voltage between identical ion-hosting electrodes. A sodium salt of sodium bis(trifluoromethylsulfonyl)imide (NaTFSI) was dissolved in distilled (DI) water (yellow dye) and an ionic liquid (red dye) of 1-Ethyl-3-methylimidazolium bis(trifluoromethylsulfonyl)imide (EmimTFSI) as shown in Fig. 1a. The two-immiscible electrolyte comprises an upper aqueous (Aq) phase and a lower ionic liquid (IL) phase. To ensure the immiscibility between Aq and IL phase, the height of the interface was monitored (Supplementary Fig. 3) and the degree of mixing was assessed using thermal galvanic analysis (TGA) (Supplementary Fig. 4)22,23,24.
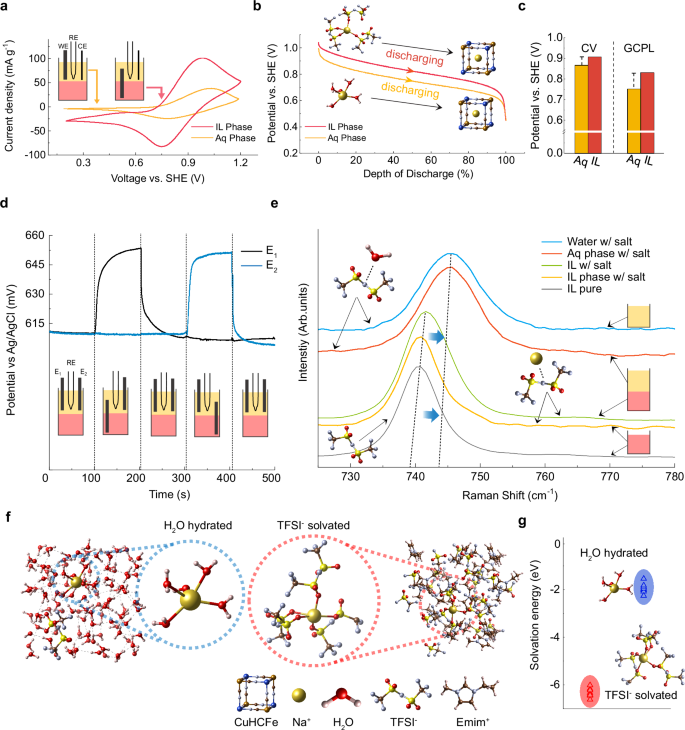
a The two-phase immiscible liquids and their solvation structures. Once Na+ based salts are dissolved into electrolytes, the corresponding molecules from the electrolytes (TFSI– and H2O) surround Na+ ions, which are called solvation and hydration, respectively. For clear visualization, Aq phase was dyed yellow, while IL phase was dyed red. b In Aq phase, 5 to 6 water molecules hydrate a Na+ ion. c In IL phase, 3 to 4 TFSI– molecules solvate a Na+ ion. d The energy diagram of various states of Na+ including intercalated into CuHCFe lattice, solvated by TFSI–, and hydrated by H2O. e The cell voltage changes between two identical CuHCFe electrodes (E1 and E2) depending on the switching of electrode surrounding. Open-circuit voltage between the electrodes was measured. The mass loading of CuHCFe is approximately 2 mg.
Depending on the solvents used, different solvation structures of Na+ ions exist25,26, with Na+ being solvated by H2O in Aq phase and bis(trifluoromethylsulfonyl)imide anion (TFSI−) in IL phase (Fig. 1b and c, respectively)27. The variations in the solvation structure for Na+ ions lead to the differences in the Gibbs free energies of solvation in Aq and IL phases, as denoted in Fig. 1d. To harness this energy, an intermediate intercalated state Na+ within an ion-hosting material is introduced28,29. In this work, copper hexacyanoferrate (CuHCFe), one of Prussian blue analogues (PBAs), is used as the ion-hosting material as CuHCFe can accommodate robust electrochemical intercalation of Na+ in both water and EmimTFSI electrolytes30,31,32,33. Owing to its fast kinetics and low voltage hysteresis, CuHCFe has been established as a prominent material for energy harvesting applications including KEH and thermal energy harvesting21,31,34,35. Additional characterizations and preliminary electrochemical tests of CuHCFe electrodes can be found in Supplementary Fig. 1 and Supplementary Fig. 2, respectively.
The intercalation of cations (in this case, Na+) into CuHCFe lattice induces a change in the Gibbs free energy of insertion, denoted as \(\Delta {G}^{i}\) (related to the electrochemical potential: \(\Delta {G}^{i}=\,-{nFE}\))36,37,38. This value is equivalent to the difference between the Gibbs free energy of solvation of the cation in CuHCFe lattice (\(\Delta {G}^{c}\)) and the Gibbs free energy of solvation of the cation in the solvent (\(\Delta {G}^{s}\)), as identified in previous studies37,39,40:
$$\Delta {G}^{i}=\,\Delta {G}^{c}-\,\Delta {G}^{s}=\,{-}{nFE}$$
(1)
where n is the number of electrons in the reaction and F is Faraday’s constant. In our experimental setup, a cell consisting of two identical CuHCFe electrodes (E1 and E2) and the two-phase immiscible electrolyte was employed (Fig. 1e). Based on Eq. (1), the cell voltage \({E}_{{cell}}={E}_{1}-{E}_{2}\) can be expressed as41,42
$${{E}_{{cell}}=E}_{1}-{E}_{2}=-\frac{\varDelta {G}_{1}^{i}\,-\varDelta {G}_{2}^{i}\,}{{nF}}$$
(2)
where \(\varDelta {G}_{\!j}^{i}\) means the Gibbs free energy of insertion of \({E}_{j}\) (\(j\)=1,2). The derivation of Eq. (2) is detailed in Supplementary Note 3 and Supplementary Fig. 33. In Fig. 1e, both electrodes were initially immersed in Aq phase from 0 s to 100 s, resulting in \({\Delta G}_{1}^{i}=\varDelta {G}_{2}^{i}\) and the cell voltage of zero. However, upon switching E1 to IL phase at 100 s, \({\Delta G}_{1}^{i}\ne {\Delta G}_{2}^{i}\). This change resulted in \({E}_{{cell}}\) becoming greater than zero, which corresponded to an increase in Ecell, which reached 45 mV during 100 s to 200 s. The cell voltage returned to zero as \({E}_{1}\) was switched back to Aq phase from 200 s to 300 s. This voltage change by switching the cation solvation can be the driving force of the electrochemical KEH.
Electrochemical potentials of CuHCFe with different solvation structures of Na+ ions
To prove the concept, we designed electrochemical characterizations with three-electrode cell configuration. In flooded-beaker cell, redox potential of CuHCFe was measured. The two-phase electrolyte and experimental scheme are described in Fig. 2a. The preparation of electrodes is explained in Supplementary Fig. 1b. CuHCFe nanoparticle was synthesized by co-precipitation method and made in the form of a slurry43. The slurry was put on a conductive glass. The glass was selected as a substrate to promote a quick switching of electrolytes. Supplementary Fig. 5 and Supplementary Fig. 6 illustrate that employing a glass-type current collector guarantees the change of solvation environment as the electrodes transition into other phases. Supplementary Fig. 2 shows the basic electrochemical characterization of CuHCFe nanoparticle electrodes in aqueous and ionic liquid electrolytes, respectively. To get cyclic voltammetry (CV) and galvanostatic cycling with potential limitation (GCPL) curves, we conducted half-cell tests with a Ag/AgCl reference electrode. As seen in Fig. 2a, CuHCFe shows different redox potentials in different electrolyte phases. The formal potentials (EF) of CuHCFe in Aq and IL phase were calculated from CV peaks (EF = (Vox + VRE)/2). In IL phase, CuHCFe exhibited a 41.2 mV higher formal potential than that in Aq phase (Fig. 2c, left). Also, from GCPL, the higher redox potential of CuHCFe electrode in IL phase was observed. In Fig. 2b, the discharging curves of CuHCFe in IL phase (red) and Aq phase (yellow) are recorded, showing a significant upper curve in IL phase. As described in Fig. 2b, in IL phase, Na+ is solvated by TFSI, while in Aq phase H2O molecules hydrate Na+. When the solvated/hydrated Na+ ions intercalate into CuHCFe structures, the solvation/hydration shells are de-solvated/de-hydrated, resulting in different redox potentials. For a fair comparison, voltages at 50% state-of-charge (SoC) in each phase were compared. When CuHCFe was half-charged, the one in IL phase exhibited a 78.9 mV higher potential than in Aq phase (Fig. 2c, right). Additionally, we also conducted Galvanostatic Intermittent Titration Technique (GITT) measurements to exclude the possibility that the increase in voltage may come from a thermodynamic fluctuation. GITT applies the use of a constant current supply and specified cut-off intervals to measure the transient voltage change and OCV drop (IR drop) during the charging and discharging processes. In Supplementary Fig. 7, GITT results are compared. Consistent with CV and GCPL, CuHCFe showed higher potential when it was in IL phase than Aq phase. From electrochemical experiments including CV, GCPL, and GITT, we can validate that the redox potential of CuHCFe in IL phase is higher than that in Aq phase.
a Cyclic Voltammetry (CV) curves CuHCFe in IL phase (red) and in Aq phase (yellow). A three-cell experiment configuration with reference electrodes was used. b Galvanostatic Cycling with Potential Limitation (GCPL) curves of CuHCFe in IL phase (red) and in Aq phase (yellow). In each liquid phase, the discharging curves of CuHCFe were obtained. The current was 0.05 C (1 C = 60 mAh g−1). c The differences in formal potential in CVs and potential at 50% state-of-charge (SoC). The values were got from (a, b). The yellow bars represent Aq phase, while the red bars represent IL phase. d The voltage profiles of two identical CuHCFe electrodes were recorded. The potential changes of E1 (black) and E2 (blue) were measured using an Ag/AgCl reference electrode. e Raman spectroscopy of characteristic TFSI– vibrational modes. From the bottom to the top, pure ionic-liquid (grey), the ionic-liquid phase with 0.2 M NaTFSI salt (yellow), the ionic-liquid with 1 M NaTFSI (green), the aqueous phase with 0.2 M NaTFSI (red), and DI water with 0.2 M NaTFSI (blue). f Atomic configurations of Na+ ions in H2O (left) and EmimTFSI environments (right) obtained from ab initio molecular dynamic (AIMD) simulations. Typical solvation structures of Na+ ions in each phase are enlarged. g Density functional theory (DFT) calculations on solvation energies of Na+ ions in Aq phase (blue) and in IL phase (red).
After investigating the redox potential of each phase, we conducted switching tests of CuHCFe electrodes (Fig. 2d). Two identical CuHCFe electrodes were charged to their 50% SoC potential in Aq phase and electrically shorted with each other for enough time to make sure there was no voltage difference between them (Fig. 2d, 0s–100s). Then one of the electrodes was switched to IL phase (at 100 s). For convenience, the electrode switched to IL phase was referred to E1 and the electrode remaining in Aq phase was referred to E2. The potentials of E1 and E2 versus Ag/AgCl reference electrode were measured. At 100 s, the voltage increased from 615 mV to 650 mV without any external power. When the increment was saturated, E1 was switched back to Aq phase (at 200 s). Then the voltage is restored to the initial value (200s–300s). At 300 s, now E2 was switched to IL phase while E1 was in Aq phase. Similar with the previous, potential of E2 increased from 615 mV to 650 mV (300s–400s) and returned to the original value (at 400 s). Furthermore, we conducted the switching test with other types of battery cathode materials in addition to CuHCFe (Supplementary Fig. 8). Lithium manganese oxide (LMO) and lithium cobalt oxide (LCO), two widely used intercalation materials, showed an increase of OCV when they were switched into IL phase. Moreover, we carried out the switching test, varying with salt cations to include LiTFSI, NaTFSI, KTFSI, and Zn(TFSI)2 as seen in Supplementary Fig. 9. Maintaining a fixed salt of 0.01 M across all cations, we observed potential increment of 108 mV, 162 mV, 20 mV, and 55 mV for Li+, Na+, K+, and Zn2+, respectively. The results of Supplementary Fig. 8 and Supplementary Fig. 9 suggest that the two-phase electrolyte system can combine various battery material types and salt types.
Throughout Supplementary Fig. 10 and Supplementary Fig. 11, we conducted experiments by varying the salt concentration to examine its effect on parameters including saturated voltage increase, saturation time, peak (short-circuit) current density, current duration period, and charge density. Our observations revealed that a lower concentration resulted in a higher voltage difference coupled with a longer saturation time, increased perk current density, extended current duration period, and elevated charge density. This was thought to be because the degree of mixing between the phases intensified as the concentration increased and decreased the voltage difference. Furthermore, the findings in Supplementary Fig. 11 suggest that by adjusting the concentration, energy harvesting parameters including output voltage, current and response time can be tuned.
Additionally, we conducted the switching experiment while varying the ionic liquids as depicted in Supplementary Fig. 12. TFSI-anion ionic liquids including N-Propyl-N-methylpyrrolidinium bis(trifluoromethanesulfonyl)imide (PYR13TFSI) and 1-Butyl-1-methylpyrrolidinium bis(trifluoromethanesulfonyl)imide (PYR14TFSI) were used alongside EmimTFSI. Upon the addition of 0.01 M NaTFSI, all ILs exhibited an increase in OCV when CuHCFe was moved into IL phase from Aq phase. Specifically, EmimTFSI, PYR13TFSI, and PYR14TFSI exhibited OCV increments of 162 mV, 27 mV, and 35 mV, respectively.
From Fig. 2a to Fig. 2d, we have verified the potential difference between IL and Aq phase in the macroscale. For a more fundamental understanding of the origin of the potential difference, we have conducted Raman spectroscopy and computational calculations. Among the components of our two-phase electrolyte, TFSI– has distinguishable peaks in Raman spectroscopy44,45. TFSI– molecule has its vibrational mode in the range from 735 cm−1 to 750 cm−1. The specific positions of TFSI– peaks are determined by the molecular bodings surrounding TFSI. In pure EmimTFSI ionic liquid, the peak appears at 740.1 cm−1 (Fig. 2e, grey). With the introduction of NaTFSI salt into the pure ionic liquid, the peak slightly shifted to the right, 741.6 cm−1, (green in Fig. 2e), which means some TFSI– are binding with Na+ ions. While, when the same amount of NaTFSI is dissolved in pure DI water, the peak shifted largely to 745.7 cm−1 (blue in Fig. 2e), indicating that TFSI– bonds with H2O molecules (vice versa: Na+ ions are also surrounded by H2O). More detailed experiments were conducted to further demonstrate the solvation structure difference. Specifically, Raman analysis on EmimTFSI, EmimTFSI + NaTFSI, IL phase, DI water, DI water + NaTFSI, and Aq phase were compiled (Supplementary Fig. 13). In Supplementary Fig. 13a, a small right peak shift ( < 1 cm−1) is observed when the salt concentration in EmimTFSI is increased from 0 to 1 M (the saturation point). Even at a saturated concentration of 1 M, the TFSI peak is at 741.6 cm−1. On the other hand, the locations of the TFSI– peaks in Aq phase are always higher than 745 cm−1 (Supplementary Fig. 13c). The results strongly supported the fact that TFSI surrounds Na+ in IL phase, while TFSI– surrounds H2O in Aq phase. From Raman analysis, we can confirm that the solvation structures of TFSI– in IL and Aq phases are different. For the next section, we verify that the difference in solvation structures makes the solvation energy difference with computational simulations.
To better understand the interplay between solvation structures and electrochemical potential in this system, we start by examining the derived equations that reflect the experimental observations. By combining Eq. (2) and the experimental results as depicted in Fig. 1e, Eq. (2) can be expressed as below:
$${E}_{{cell}}={E}_{{IL}}-{E}_{{Aq}}=-\frac{\left(\varDelta {G}_{{IL}}^{c}\,-\varDelta {G}_{{IL}}^{s}\right)-\left(\varDelta {G}_{{Aq}}^{c}\,-\varDelta {G}_{{Aq}}^{s}\right)}{{nF}} \, > \, 0$$
(3)
In Eq. (3), \({G}^{c}\) denotes the Gibbs free energy of the cation solvation within CuHCFe lattice, whereas \({G}^{s}\) signifies the Gibbs free energy of the cation solvation in the solvent. By decomposing Eq. (3) into terms representing solvation within CuHCFe and in the solvent, it can be expressed as follows:
$$\left(\varDelta {G}_{{IL}}^{c}\,-\varDelta {G}_{{Aq}}^{c}\right)+\left(\varDelta {G}_{{Aq}}^{s}-\varDelta {G}_{{IL}}^{s}\right) < 0$$
(4)
In Eq. (4), the first term, \((\varDelta {G}_{{IL}}^{c}\,-\varDelta {G}_{{Aq}}^{c})\) represents the Gibbs free energy difference between solvation of the cation in CuHCFe lattice in each solvent. The second term, \((\varDelta {G}_{{Aq}}^{s}-\varDelta {G}_{{IL}}^{s})\) represents the Gibbs free energy difference between solvation of the cation in each solvent. Equation (4) further refines our understanding by quantifying the interplay between Gibbs free energy changes in the solvent and CuHCFe situations. Their combined sum must be negative to explain the sign of the voltage observed in experiments (Fig. 1e).
To investigate how Na+ ions interact with surrounding solvents and to initially assess \(\Delta {G}^{s}\), ab initio molecular dynamics (AIMD) simulations were employed. These simulations were performed on the atomistic configurations of 0.5 M NaTFSI/H2O and NaTFSI/EmimTFSI electrolytes, which are our model systems for Aq phase and IL phase, respectively (see Computational Details for further information). Figure 2f shows the characteristic solvation structures in Aq phase and IL phase obtained from the AIMD results. Upon examining the solvation structures, we observed that Na+ associates with 5 to 6 H2O molecules (Aq phase) and 3 TFSI– anions (IL phase). In each phase, the different surrounding environments can lead to variations in the strength of interactions between Na+ and the solvation structures (Supplementary Figs. 14 and 15). These variations are expected to significantly influence the \(\Delta {G}^{s}\) values acting as key factors in the energy differences observed when Na+ desolvates from these structures46,47,48. To quantitatively compare these effects and understand their impact on \(\Delta {G}^{s}\), solvation energy was approximated using the first solvation shell49. The solvation energy of Na+ ions in the solvent is written as Eq. (5)
$${Solvation}\; {energy}\; {of}N{a}^{+}{in\; the\; solvent}= {E}_{{{{Na}}^{+}({H}_{2}O{or}{{TFSI}}^{-})}_{n}}\\ -{E}_{{({H}_{2}O{or}{{TFSI}}^{-})}_{n}}-{E}_{{{Na}}^{+}}$$
(5)
where \({E}_{{{Na}}^{+}}\), \({E}_{{({H}_{2}{O\; or}{{TFSI}}^{-})}_{n}}\), and \({E}_{{{{Na}}^{+}({H}_{2}{O\; or}{{TFSI}}^{-})}_{n}}\) are DFT energies of a Na+ ion, a (H2O or TFSI–)n cluster, and a Na+(H2O or TFSI–)n cluster in a vacant simulation box, respectively (Supplementary Table 1). Structures with various solvation structures were sampled at 5 ps intervals during 50 ps in AIMD simulations, resulting in the extraction of ten solvation structures for each phase. The solvation energies in solvents were then calculated, and the results are presented in Fig. 2g. Since IL phase exhibits more negative energies compared to Aq phase, this leads to a positive \((\varDelta {G}_{{Aq}}^{s}-\varDelta {G}_{{IL}}^{s})\).
Given that the solvation energy results indicate \((\varDelta {G}_{{Aq}}^{s}-\varDelta {G}_{{IL}}^{s})\) is positive, we can infer that \((\varDelta {G}_{{IL}}^{c}\,-\varDelta {G}_{{Aq}}^{c})\) might be negative to ensure the combined sum adheres to the requirements of Eq. (4). Differences in \(\Delta {G}^{c}\) across both phases may be attributed to the distinctive intercalation mechanisms of Na+ ions. In Aq phase, Na+ ions typically undergo partial desolvation with 2−3 H2O molecules during intercalation into the CuHCFe, forming Na+ + H2O clusters (Supplementary Fig. 16)30,35,36,40. Conversely, in IL phase, Na+ ions intercalate independently. Considering the size of the TFSI– anion (length: 1.132 nm and width: 0.838 nm)50 relative to the CuHCFe’s intercalation site (341 pm)38, it is unlikely that TFSI– solvated Na+ would intercalate along with the TFSI–. To further explore these trends, we defined and calculated the solvation energy of Na+ within the CuHCFe as a proxy to elucidate the correlation with \(\Delta {G}^{c}\). Our findings indicate that in Aq phase, an increase in the degree of partial desolvation of the Na+ ion with H2O correlates with an increase in positive values of solvation energy, suggesting a negative trend in \((\varDelta {G}_{{IL}}^{c}\,-\varDelta {G}_{{Aq}}^{c})\) (Supplementary Fig. 17). Additionally, the reduction in the positive values of \((\varDelta {G}_{{Aq}}^{s}-\varDelta {G}_{{IL}}^{s})\) (Supplementary Fig. 18), resulting from partial desolvation in Aq phase, aligns more closely with the voltage sign trends observed in experiments, as described by Eq. (4). Consequently, to optimize the voltage in this system, it is imperative to take into a broader spectrum of factors (Supplementary Note 2, Supplementary Fig. 19 and Supplementary Fig. 20) beyond solvation energy alone, recognizing the complex interplay of solvation dynamics and intercalation effects.
From the experimental results of electrochemistry, spectroscopy, and molecular simulation, we can clarify the origin of voltage increment. In the next chapter, we explain how we can make KEH cycles by using the voltage increment.
Kinetic energy harvesting cycles and performances
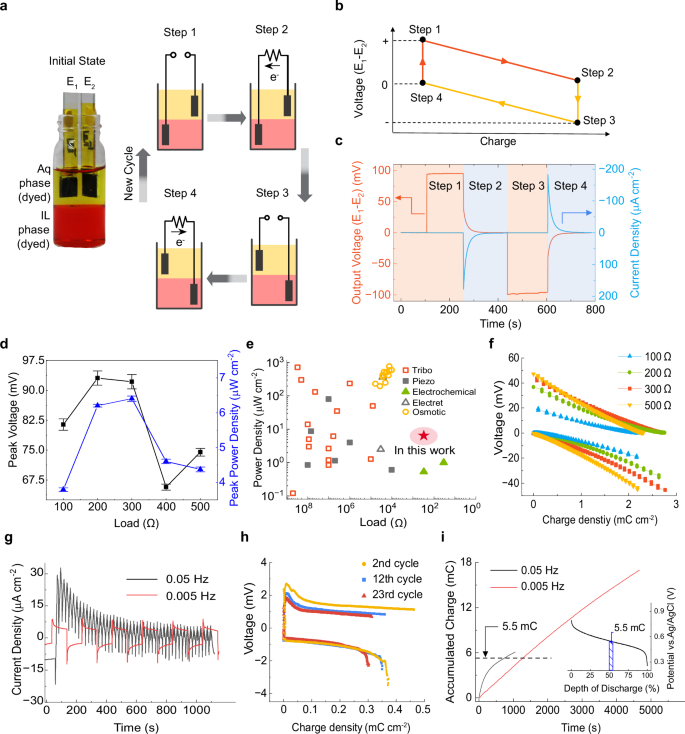
The KEH using the solvation energy difference in the two-phase electrolyte is demonstrated by the alternating switching of two CuHCFe electrodes between the two phases. Figure 3a presents the operational mechanism of the electrochemical system of KEH modulated by solvation energy, while Fig. 3b presents its corresponding cycle diagram. Two identical CuHCFe electrodes (E1 and E2) are charged in Aq phase up to 50% SoC and then electrically shorted to make the equilibrium between them. After sufficient time for shorting, E1 is switched to IL phase in step 1. Due to the difference in the solvation states, E1 acquires a higher potential than E2. When an external resistor is connected in step 2, current flows from E1 to E2, reducing the voltage to zero. As the current flows, E1 undergoes reduction, while E2 undergoes oxidation. As a result, Na+ ions are intercalated into E1 and released from E2. In step 3, E2 is switched to IL phase while E1 is moved back to Aq phase. The potential difference occurs again but in the opposite direction. In step 4, current flows from E2 to E1, causing E2 to be reduced and E1 to be oxidized, ultimately leading to the system returning to its initial equilibrium state. The continuous oxidation/reduction of the electrodes was induced by mechanical switching of their positions. The kinetic energy required for the switching was harvested into electricity without any external voltage bias.
a Energy harvesting cycle of switching two CuHCFe electrodes alternatively into Aq/IL phases. b The schematic draw of voltage versus charge plot during a harvesting cycle. The amount of energy harvested through a cycle is the area enveloped in the loop. In step 1 and step 2, a positive voltage is obtained, while a negative voltage is obtained in step 3 and step 4. c The profiles of output voltage (red) and current density (blue) during a cycle. An external load of 300 Ω was connected. d Impedance matching experiments. While changing the values of resistor load, peak voltage (black), and peak power density (blue) were measured. The error bars represent the standard deviation. e Comparing plots among KEH methods. The full descriptions of each component are presented in Supplementary Table 4. f Voltage versus charge loop curves in a cycle. Voltage and charge were calculated based on the resistance and the current measured. The symbols represent different resistor loads: blue triangle for 100 Ω, green circles for 200 Ω, red rectangles for 300 Ω, and yellow reverse triangles for 500 Ω. g Profiles of current density with the switching frequency of 0.05 Hz (black) and 0.005 Hz (red). h Voltage versus charge loop curves at 0.005 Hz. The second (yellow circles), 12th (blue rectangles), and 23rd (red triangles) cycles are compared. i The amount of accumulated (moved) charge during cycles for long-term cycles at 0.05 Hz (black) and 0.005 Hz (red) is shown. The amount of charge by self-discharging is displayed in the inset. Through all experiments, CuHCFe nanoparticle slurry on ITO-coated glass electrode was used with a mass loading of approximately 2 mg of CuHCFe.
Figure 3c presents the experimental voltage and current profiles for the energy harvesting cycle at an external load of 300 Ω. The experimental setup and switching procedures are described in Supplementary Movie 1. Open-circuit voltage (OCV) was measured during steps 1 and 3, whereas the output voltage was computed based on the current flowing the load during steps 2 and 4. The peak voltage achieved was 96 mV of OCV, accompanied by a peak current density of 183 μA cm−2. Following its peak value, the current exhibits a decay, reaching half of its maximum value within 10s−15s. Subsequently, the remaining half of the initial current undergoes a gradual decay, dwindling to zero over the next 180 s. Figure 3d shows the peak voltages and peak power densities as a function of varying load resistances from 100 Ω to 500 Ω. The peak voltage increases as the load increases until 200 Ω with a maximum of 92 mV and decreases as the load increases. The peak power density (P) was calculated based on P = I2R, where I is the current density through the load R. The peak power density increases as the load increases until 300 Ω with a maximum of 6.4 μW cm−2 and decreases as the load increases. Furthermore, electrochemical impedance spectroscopy (EIS) analysis was performed to determine the system’s impedance, as depicted in Supplementary Fig. 21. A plot of comparison with other KEH methods in regard to output power density versus impedance is in Fig. 3e. Nanofluidic or osmotic energy harvesting (OEH) seems promising in terms of their relatively lower impedance ( < 100 kΩ) and comparable power density ( ~ 100 μW cm−2). Despite those strengths, studies on OEH devices only focus on tiny areas of active materials. The advantage of the electrochemical-based KEH method, including our present study, is low impedance which is attributed to the use of liquid electrolytes and ions. Our research improved the low power output, which was a problem in other electrochemical systems, by more than 10 times19,21. P/TENGs show the highest power due to their high output voltage. However, their high impedance inhibits the growth of the market needs. To overcome the disadvantages of high impedance, friction-based devices often embed with additional signal processing circuits for compensating their high impedance, which hinders miniaturization them51,52. Therefore, our system may be suitable as a power supply for compact and space-constrained devices.
Figure 3f converts the result given in Fig. 3c and various loads into loop curves of the output voltage for the flown charge produced by one cycle of harvesting. The harvested energy density per cycle (E) was calculated based on E = QV where Q is the charge density and V is the voltage. For 100, 200, 300, and 500 Ω, the harvested energy density per cycle is 45, 95, 116, and 99 μJ cm−2, respectively. Compared with a recent study on the electrochemical method for KEH21, our system has at most 110 times higher energy density. Moreover, for loads of 100, 200, 300, and 500 Ω, the surface charge density per cycle is 2.17, 2.64, 2.73, and 2.18 mC cm−2. The higher harvested energy and charge transfer comes from the longer duration time ( > 150 s) of current flow as aforementioned in Fig. 3c. Supplementary Fig. 22 illustrates the (surface) charge density of KEH approaches, with a particular focus on electrochemical methods, TENGs, and PENGs. Among TENGs, the highest charge density achieved to our best knowledge is reported by ref. 53 at 0.88 μC cm−2. It is notable that conventional KEH approaches typically do not surpass 1 μC cm−2. Conversely, electrochemical approaches, including our studies, exhibit charge densities that are orders of magnitude higher than those of P/TENGs. Specifically, our system recorded charge densities of 2.73 mC cm−2, 300 μC cm−2, and 240 μC cm−2 for figs. 3f, h, 4e, respectively. These results are marked as ‡, †, and *, respectively. Notably, our system achieved the highest charge density of 2.73 mC cm−2 surpassing Ref. 53 by 104 orders of magnitude. The high charge density in our system can be attributed to the utilization of battery material CuHCFe, which exhibits a specific capacity of 60 mAh g−1.
a An illustration of the microfluidic harvester. b An illustration of the inside of the microfluidic channel, which consists of the two-phase electrolyte and two CuHCFe thin-film electrodes. Upon pressure, the liquid train moves back and forth. c Optical microscopic images of microfluidic channel with CuHCFe electrodes. The energy harvesting cycle is indicated step-by-step. The scale bar in Step 3, 4 is 500 μm. The details on the fabrication process are in Supplementary Fig. 24. d Profiles of the output voltage (red) and current density (blue) of the microfluidic harvester. A load resistor of 10 kΩ was connected, and the current through the load was measured. e Voltage versus charge loop-curve for a harvesting cycle with a load of 10 kΩ. f Power density curves of the microfluidic harvester with a load of 10 kΩ. The deposition process of CuHCFe thin-film can be found in Methods.
Conventional KEH methods have relied on the utilization of high-frequency and periodic vibrations54. However, the applications of these technologies are limited due to the non-periodic and infrequent nature of kinetic energy present in environments52. By accommodating low-frequency input, KEH devices can become more versatile and widely applicable. This is crucial for various emerging technologies, such as wearable and implantable devices, environmental monitoring systems, and agricultural sensors. Low-frequency switching experiments were conducted at 0.05 Hz and 0.005 Hz (Fig. 3g) connected to a load of 300 Ω. The two electrodes were switched to the other phase every 10 s or 100 s, respectively. Thus, one cycle requires 20 s or 200 s, i.e., 0.05 Hz or 0.005 Hz, respectively. In the 0.005 Hz curve (Fig. 3g, red), the current density reaches a maximum value of approximately 8 μA cm−2 and maintains a steady current output of 3–5 μA cm−2 for 100 s. The complete cyclic results of 0.005 Hz for a duration of 5000 s exhibited consistent harvested energy per cycle as presented in Supplementary Fig. 23. Figure 3h converts the result given in Fig. 3g into loop curves of the output voltage for the flown charge produced per each cycle at harvesting 0.005 Hz of kinetic energy. The harvested energy densities were calculated similarly with Fig. 3f. For the second, twelfth, and twenty-third cycles, the harvested energy densities are 0.882, 0.68, and 0.54 μJ cm−2. The decreased energy densities per cycle compared with Fig. 3h come from the insufficient time for CuHCFe electrodes to reach their equilibrium.
It is crucial to check if the electrochemical energy harvesting system discharges its energy in the harvesting demonstration and overestimates the harvesting performance. To eliminate the possibility of the overestimation of the kinetic energy harvesting due to the self-discharge of the two electrodes, we compared the charge capacity of CuHCFe electrode before and after the energy harvesting cycle and the accumulated charge during harvesting cycles. Figure 3i shows the accumulated amount of charge when the frequency of kinetic energy is 0.05 Hz (black) or 0.005 Hz (red). 250 cycles at 0.05 Hz for 1000 s and 25 cycles at 0.005 Hz for 5000 s were operated. There was no additional charging on the electrodes during the whole process. We compared the potential of the CuHCFe electrodes with a Ag/AgCl reference electrode before and after the long-term cycle, and the resulting voltage drop was 15 mV. The charge amount associated with the voltage drop was then calculated and found to be 5.5 mC as seen in Fig. 3i (inset). It is worth noting that this charge is significantly smaller than the accumulated charge during the cycle, indicating that most of the electricity was derived from energy harvesting instead of the self-discharge of the electrodes.
Microfluidic kinetic energy harvester
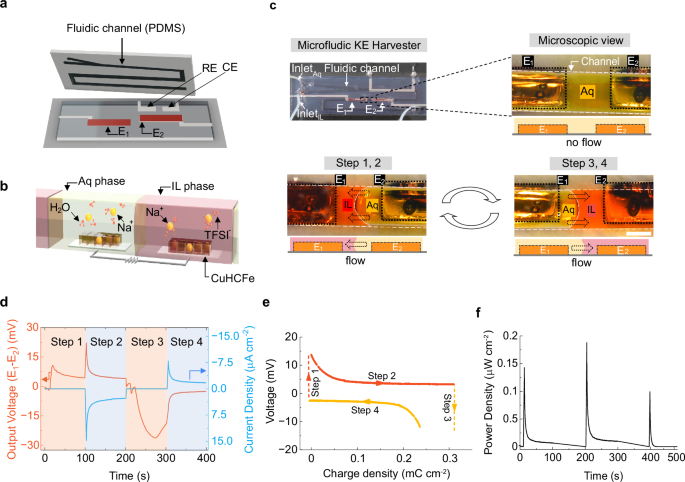
For the practical demonstration, the aforementioned flooded-beaker experiments were transformed into a kinetic energy harvester inspired by microfluidic devices. In the microfluidic device, we employed the same principle of manipulating ion solvation as in the previous flooded-beaker cell experiments, where redox reactions facilitated the conversion of kinetic energy to electricity. However, the key difference is that the kinetic input moves the electrolyte in the microfluidic channel instead of electrodes. A microfluidic kinetic energy harvester consists of the identical two CuHCFe thin-film electrodes facing the substrate and the two-phase electrolyte inserted into a microfluidic channel. Figure 4a, b display the 3D design of the device and inside of the channel, respectively. The suitability of CuHCFe thin-film foam for micro-sized devices was demonstrated in a previous work55, while its deposition process was found to be compatible with conventional photolithography techniques. To facilitate the adoption of microfluidic devices, a pair of identical CuHCFe thin-film electrodes were deposited on the substrate to enable their placement in separate Aq and IL electrolytes, respectively. The details of CuHCFe thin-film deposition and fabrication processes of microfluidic devices can be found in Methods and Supplementary Fig. 1, Supplementary Fig. 24, and Supplementary Fig. 25. The narrow dimensions of the fluidic channel facilitated horizontal phase separation of Aq/IL phase, enabling the creation of a serial liquid-train of the two-phase electrolyte running on the surfaces of CuHCFe electrodes as illustrated in Fig. 4b. Thanks to the low Reynolds number in a microfluidic channel (Supplementary Fig. 26), each liquid phase formed laminar flows on the CuHCFe electrodes without breaking the interface.
A photograph of a single-pair microfluidic harvester and the microscopic images of the fluidic channel are presented in Fig. 4c. The working principle of harvesting kinetic energy using the flow of electrolytes is illustrated step-by-step. Initially, two CuHCFe electrodes (E1 and E2) are in contact with Aq phase, and the voltage between them was zero. In step 1, Aq/IL two-phase liquid train was set in motion by an input kinetic energy, causing E1 in contact with IL phase and E2 with Aq phase. In step 2, electric current flow through the connected external load between E1 and E2. During the process, E1 and E2 undergo reduction and oxidation, respectively. Once the voltage is dropped to zero at the equilibrium, the kinetic input propelled the liquid train once again, causing Aq phase to cover E1 and IL phase to cover E2. As the electrode in IL phase had a higher potential, the voltage between E1 and E2 rises again but in the opposite direction (step 3). In step 4, as the current flows, the voltage gradually decreases to zero at another equilibrium. Meanwhile, E1 is oxidized and E2 is reduced. As a result, after a complete cycle of energy harvesting, the electrodes are returned to their initial states.
In Fig. 4d, the voltage and current profiles obtained during a cycle of energy harvesting in the microfluidic harvester are presented. The current was measured with an external load of 10 kΩ. During step 1 and step 3, we measured OCV, while in steps 2 and 4, the voltage was calculated based on the current flowing through the load. The peak voltage achieved was 20 mV, and the peak current density was 15 μA cm−2. Figure 4e presents the result given in Fig. 4d into loop curves representing the output voltage for the flown charge generated by one cycle of harvesting. With connected to a load of 10 kΩ, the maximum peak power density was recorded as 0.2 μW cm−2 as seen in Fig. 4f. During the cycle, the harvested energy amount is 1.68 μJ. To evaluate the energy conversion efficiency from kinetic to electric energy, a numerical analysis was performed using COMSOL Multiphysics. The specifics of the efficiency calculation are outlined in Supplementary Fig. 27, Supplementary Fig. 28, and Supplementary Fig. 29. In the simulation, a single phase of laminar flow was considered for both water and EmimTFSI, respectively. The energy conversion efficiency is estimated to range from 0.37% to 88.1%. Given that capillary force and surface tension were not considered, the actual value of the efficiency may be lower than our estimate.
The integration of multiple arrays of electrodes in our harvester can achieve the systematic generation of high voltage. Leveraging the versatility of microfluidic device design, we implemented a configuration with multiple (8-pair) CuHCFe electrodes. The 8-pair microfluidic harvester is described in Supplementary Fig. 30, and the short-circuit current of the 8-pair harvester is presented in Supplementary Fig. 31. Although not explored in this paper, the introduction of an insulating liquid, such as silicone oil, following Aq and IL phases, would act as a barrier, preventing electrical contact between each array of electrodes. This strategy would enable the series connection of multiple arrays, leading to the achievement of high voltage as illustrated in Supplementary Fig. 32a. As an alternative method to validate the increase in voltage output through a series connection, we conducted a series connection experiment using a flooded beaker-cell setup, as depicted in Supplementary Fig. 32b, c. To achieve this, we assembled 10 cells comprising Aq/IL two-phase electrolytes and two CuHCFe electrodes each. Upon arranging them in series, the resulting voltage was measured at 935 mV, which was sufficient to power a calculator, as demonstrated in Supplementary Movie 2.